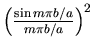Method

We want to find the intensities of each peak in the diffraction pattern, to see if the data match the theoretical expectation of equation (4), and if they do, to extrapolate the zeroth order flare intensity. But because the dots become wider and even split into double images (see Section 2), we cannot just measure the highest pixel in each peak. Therefore, we summed up the intensities of all the pixels in each bright spot, subtracting from each pixel the mean background intensity near each bright spot to ignore other light coming from the sun unrelated to the diffraction pattern.

The March 15 flare is dimmer and its shape is more nebulous. To reduce errors coming from measuring intensities over different sized areas for different peaks, we fixed the number of pixels for each order at 33. Dispersion has only a small effect because we examined only the first three orders. Otherwise, we measured the intensities by the same method. The zeroth order also has to have the background taken into account, because any intense background around the zeroth order of the flare will also be diffracted to become background around the higher orders.

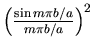To find the value of $b/a$, we analyzed the data to see where the intensity of the peaks drops to zero. Since the term $\left(\frac{\sin m\pi b/a}{m\pi b/a} \right)^{2}$ is what modulates the peaks, that term would go to 0 when the sine goes to zero, that is, when $mb/a$ is a whole number. We could then find what value of $b/a$ would make $mb/a$ an integer exactly when the intensity of the peaks drops to 0.

The highest pixel of each order, $I_{max}$, will have a certain ratio to the full intensity of the order, $I_{tot}$, and it will decrease as the order increases and the dots become wider. To estimate how $I_{max}(0)$ relates to $I_{tot}(0)$, we found $I_{max}$ for each order and compared it to $I_{tot}$.